Phase, Gain, Position, and Polarization Calibration
Coordinate Systems at the VLA
The VLA supports the J2000 coordinate system. Details on this implementation are given by Barry Clark in VLA Computer Memorandum 167 of May 11, 1983. The complete description of the J2000 coordinate system can be found in the USNO circular 163 edited by G. H. Kaplan of the Naval Research Laboratory. See also Johnston et al 1995 (AJ 110, 880). In summary, positions given in the J2000 system will be precessed in accordance with the recommendations of USNO 163. Positions given in the B1950 coordinate system will employ adjustments, so that they are effectively processed by the recommendations of the Explanatory Supplement of 1960. Positions of any other epoch will, currently, be precessed by the recommendations of the Explanatory Supplement of 1960. The most serious consequence of this is that planetary coordinates given in apparent coordinates of observing date are assumed to be in the system of the FK4. It seems likely that as soon as the system of FK5 comes into greater use for the production of planetary ephemerides, we shall reverse this decision, and use the FK5 and the formulae of USNO 163 for epochs other than 1950.
The B1950 system used at the VLA is a bit of an historical oddity. The VLA needed calibrators with accurate positions before the various astrometric (VLBI) lists existed, so they were measured using a reference frame based on a handful of positions (of order 10) from observations with the Green Bank three-element interferometer in 1979.9; those sources were therefore all referred to the B1950 system, epoch 1979.9. When the astrometric lists started coming out from JPL and Goddard, NRAO both added new sources and replaced the old positions with the new, more accurate measurements; these are in the J2000 system, precessed to epoch 2000.0. So the VLA has two fundamental systems: the B1950, epoch 1979.9 frame most natural for the old calibrators, and the J2000, epoch 2000.0 frame most natural for the new ones. In transforming from one frame to the other, e.g. to get a B1950 position for a source originally measured in J2000, both the VLA on-line computers and the OBSERVE program which creates the VLA schedule are "hard-wired" to precess between (B1950, epoch 1979.9) and (J2000, epoch 2000.0). All positions measured at the VLA are in one of these two frames. Just recently positions from the reference frame of Eubanks 1995-1 have been adopted for 475 sources in the calibrator manual, replacing many less accurate positions, and bringing the VLA and VLBA onto the same reference frame.
It now seems profitable for anyone interested in the highest positional accuracy to use J2000 coordinates for all future observations, unless compatibility with previous observations is critical and the whole series does not span enough time to be unduly confused by the known error in Newcomb's precession constant. (It is probably less work to put previous observations in J2000 coordinates than it is to calculate the corrections to the 1950 coordinates of various dates, if the observations span more than a couple of years). For the general VLA user, the pressure to change systems is not so strong--he/she must consider whether it is more important that his observation remain compatible with previous observations of the object, or whether it should be compatible with future accurate astrometry, either radio or optical. We do not recommend changing to J2000 coordinates for an object which you have observed here before, and might conceivably wish to combine the old (u,v) data--there is no point in just asking for trouble. However, we encourage the use of J2000 coordinates for new observations. It seems inevitable that J2000 coordinates are going to come into general use, and the sooner we can get through the painful transition period, the better off we all shall be.
Hints and Strategies for Successful Phase Calibration
In most programs, calibrator sources are observed at least once an hour and sometimes as frequently as every 10 minutes. Calibrator observations are not only important for tracking instrumental phase and gain drifts, atmospheric and ionospheric gain and phase variations, but for monitoring the quality and sensitivity of the data and for spotting the occasional gain and phase jumps.
There are several criteria for choosing and using a calibrator. A list of guidelines, in decreasing order of importance, follows:
- Choose the calibrator closest to your source. If it is within 10 deg., atmospheric phase fluctuations will be somewhat better calibrated. It is better to have one calibrator per source over the entire run. If several are needed, try to bootstrap their positions together. However, in the smaller configurations and at longer wavelengths, these criteria can be considerably relaxed, so a single calibration for a group of sources is often preferable. Furthermore, if your target sources can be self-calibrated, the need for rapid switching between source and calibrator is entirely removed. Hourly observations of the calibrator are more than sufficient for this case, except at 22 GHz or higher frequencies where they should be no further apart than 30 minutes
- Choose a calibrator which has a P or S quality status for the desired configuration and frequency (see Section 4.1). The difference between P and S is minimal but P is preferred since fewer gain errors will result. However, a more nearby but weaker S or even W quality calibrator may well be preferable for phase calibration, but not for amplitude calibration. In this case the amplitude calibration, which is much more stable than the phase calibration, can be derived from observations of a more distant P quality calibrator that is observed less frequently. This situation may arise at high frequencies where only a small number of sources are sufficiently strong (> 0.5 Jy) for amplitude calibration, but the atmospheric phase fluctuations require a nearby calibrator source. As a general rule of thumb, at 0.7 cm the phase calibrator should be within 10 degrees in good weather and within 5 degrees in bad weather. If just solving for the phase, the calibrator can be as weak as twice the sensitivity on a single baseline, which is 0.1 Jy at 43 GHz. If no VLA calibrator is sufficiently close, it may be useful to consult the MERLIN calibrator lists of Patnaik et al. (1992, MNRAS, 254, 655) and Browne et al. (1998; MNRAS, 293, 257). And to properly remove tropospheric phase fluctuations at high frequencies requires very rapid switching with observations of the calibrator every few minutes.
- At frequencies of 1.8 GHz and below, the presence of moderately strong sources within the primary beam centered on the calibrator can cause significant closure errors. For this reason many calibrators have uv restrictions at L and P band and may be completely unsuitable in the smaller configurations. Observations performed in spectral line mode may encounter somewhat larger closure errors than indicated by the P or S quality flags (see the Key in section 4.1) due to the reduction in bandwidth smearing. When observing at L band in the D and C configurations it may be desirable to choose a calibrator with P quality status, even if it is more distant from the target source. Fortunately the atmosphere is quite stable at L band in the D and C configurations.
- Different calibrator codes are used only to distinguish the accuracy of the calibrator position. If absolute positional accuracy < 0.1 arcsec is desired, the position code should be an important consideration - use 'A' or 'B' calibrators. Most positions for sources with 'A' or 'B' PC codes are taken from the JPL or USNO astrometric lists.
- The flux density of the calibrator is of secondary importance. The only exceptions are when the calibrator will be used as a band-pass calibrator for spectral line observing, for high dynamic range observations where closure errors must be measured, and for very narrow-band spectral line experiments.
- The use of partially resolved calibrators for the determination of antenna gains and phases is possible with the added complication that the calibrator must be imaged first and the resulting model provided to CALIB. Use of partially resolved calibrators may occasionally be necessary in the larger configurations. Models are available on the web at http://www.aoc.nrao.edu/~cchandle/cal/cal.html
Planning for Good Polarization Calibration
Introduction
When observing in any of the continuum modes the cross hand products (RL and LR) are produced automatically by the correlator for each IF. Typical "impurities" of the feeds are about 5% for the center of most VLA bands and degrade toward the band edges and away from the pointing center in the image plane. Without any polarization calibration an unpolarized source will appear to be polarized at the ~1% level. Furthermore, without calibration of the R-L phase difference, the polarization angle is undetermined. Fortunately it is not difficult to obtain a reasonably good polarization calibration under most circumstances. With a modest investment of time spent on calibrators and a little effort the instrumental polarization can be reduced to less than 0.1%.
Determining the Leakage Terms (aka D-terms)
The best way to determine the leakage terms (which produce the instrumental polarization), is to observe an unresolved source over a wide range in parallactic angle. The polarization of the calibrator will appear to rotate in the sky with parallactic angle while the instrumental contribution stays constant. The AIPS task PCAL uses this behavior to simultaneously solve for the source polarization properties and the leakage terms. The usual recommendation is for 5 or more observations covering 100 degrees or more of parallactic angle in roughly uniform steps. Strong and compact calibrators (code "P") sources are preferred, although often a reasonably good solution can be obtained from a weaker calibrator observed many times throughout a run for gain and phase calibration. More than one source can be used in the solution provided all sources are unresolved. Algorithms have also been developed in AIPS recently (for VLBI polarization calibration) that allow the use of a single resolved calibrator. In planning an observing run it may be useful to consult the parallactic angle diagram below:
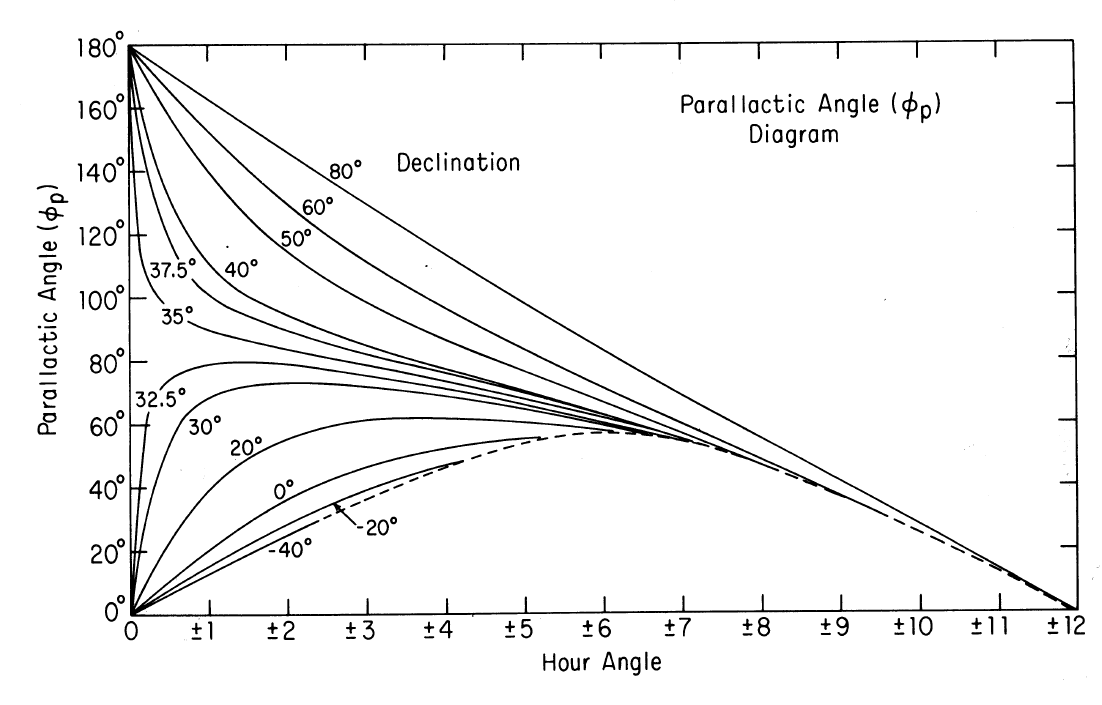
Figure - Parallactic Angle vs. hour angle for various declinations
The leakage terms are also known to be time-variable (Holdaway, Carilli & Owen 1992, VLA test memo #163) and software has been developed in AIPS++ to contend with this variability.
A single observation of a strong unpolarized source (or a source with well known polarization properties) can be used to determine the leakage terms. As an example, 3C84 is strong, unpolarized and unresolved for most VLA frequencies and configurations, so that a single scan on 3C84 may be sufficient. The leakage terms are also fairly constant over weeks to months, so that measurements from one observing run can be passed to another made using the same frequencies and bandwidths and observed in the same configuration. The leakage terms are carried entirely in the antenna table, so to transfer them one merely copies the antenna table.
Calibrating the absolute polarization angles
Calibration of the absolute polarization angle (or R-L phase difference) can be accomplished with a single observation of a polarized source having a known polarization angle (the true R-L phase difference will be twice the source polarization angle). The best source for these purposes is 3C286, which is also a primary flux density calibrator. If 3C286 can't be reached then 3C138 will work in most circumstances. Some information is known about 3C48 and 3C147 as well. All observations of polarization angles summarized below are tied to 3C286 which is assumed to have a Faraday Rotation Measure of 0 rad m$^{-2}$.
| 1995.2 Polarization Measurements | ||||||||||||
| Showing R-L Phase Difference (degrees) and Fractional Polarization (%) | ||||||||||||
| Source | 20cm | 6cm | 3.7cm | 2cm | 1.3cm | 0.7cm | ||||||
| 3C48 | N/A | 0.5 | -148 | 4.1 | -132 | 5.3 | -135 | 7.0 | -145 | 7.6 | -166 | 9.2 |
| 3C138 | -18 | 7.9 | -22 | 11.1 | -22 | 11.9 | -24 | 10.8 | -30 | 10.6 | -23 | 11.6 |
| 3C147 | N/A | <0.1 | N/A | <0.1 | -57 | 0.8 | 110 | 3.1 | 145 | 4.2 | 180 | 5.2 |
| 3C286 | 66 | 9.4 | 66 | 11.0 | 66 | 11.7 | 66 | 12.0 | 66 | 12.0 | 66 | 12.5 |
| 1999.2 Polarization Measurements | ||||||||||||
| Showing R-L Phase Difference (degrees) and Fractional Polarization (%) | ||||||||||||
| Source | 20cm | 6cm | 3.7cm | 2cm | 1.3cm | 0.7cm | ||||||
| 3C48 | -60 | 0.4 | -148 | 4.1 | -138 | 5.6 | -134 | 7.0 | -146 | 8.2 | -172 | 8.8 |
| 3C138 | -15 | 8.0 | -20 | 11.4 | -22 | 11.7 | -24 | 11.7 | -30 | 11.6 | -28 | 12.2 |
| 3C147 | N/A | <0.1 | 16 | 0.4 | -54 | 0.7 | 109 | 2.9 | 147 | 4.5 | 170 | 6.5 |
| 3C286 | 66 | 9.4 | 66 | 11.2 | 66 | 11.6 | 66 | 12.1 | 66 | 12.4 | 66 | 13.3 |
No ionospheric correction has been made to these data, and fluctuations in the ionospheric Faraday rotation, especially approaching the solar maximum in 1999, could cause an error in the above determinations by 10 degrees or more at 20cm. As 3C48 is only weakly polarized at 20cm, it should not be used at this frequency. If it must be used (e.g. no suitable calibrator was observed) then the polarization angle given at epoch 1999.2 can be used. 3C48 may also be undesirable at 22 and 43 GHz where it is weak and variable. At 90cm all the above sources are unpolarized. Some pulsars, however, are strongly polarized and we are investigating using these along with good ionospheric models and data to obtain polarization calibration at 90cm. Contact Rick Perley (rperley@nrao.edu) for further information.




Connect with NRAO